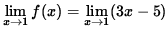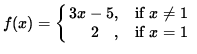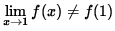Heat Miser

1. The four places where derivatives do not exist is:

      f(x) = abs(x) It is a corner.

     A cusp.

   A jump in a graph, like a piecewise function.

    An asymptote, such as in a rational function.

2. Implicit Differentiation- The first step is to find the derivative of each part of the equation. 

After finding each variable, put the equation over dx. Ex: 2y= 2*dy/dx.

Solve for dy/dx by getting all of the variables of that type on one side.

Divide to get dy/dx by itself.

3. The most important thing to remember in a related rate problem is to write the dy/dx in the right spot. If it's put in the wrong spot or not included then the whole problem is incorrect.

No Ceilings

1. f'=0 represents the vertex of the original equation because the slope(derivative) is zero, so it is not increasing or decreasing.

2. You can identify where a function increases when you calculate the derivative in front of the vertex and the point is less than the point of the vertex(or critical point). You can tell where it's decreasing if the derivative after the vertex is less than after the vertex(or critical point). If the derivative is negative, the equation is decreasing. If it is positive it is increasing.

3. The chain rule is the process of finding the derivative of a composite function.
 If f(x)=g(h(x)) then f'(x)=g'(h(x))*h'(x)
In reality all your doing is...
   1. Taking the derivative of the outside function
   2. Rewriting the interior function
   3. Multiplying by the derivative of the inside

Find the derivative and the equation of the tangent line where x=2.

f(x)= (2x^2 - x)^2      f'= 2(2x^2 - x) * 4x-1     =      2(4x-1)(2x^2 - x)
(8x^3 - 6x^2 + x)2     f'=16x^3 - 12x^2 + 2x 

Point: (2, 36)     Slope:84         Equation: y= 84x + 204

4. h(x)= f(g(x))      g(-4)=5    g'(-4)=2       f'(5)=20      Find h'(-4).

Calculus Continued...

1. Where continuity does not work

The limit is approaching 1. When it is 1 it equals 2. When it's not 1, but very very close, it equals -2(plugged into the 3x-5 equation). So the limit is not continuous at 1 because the two equations do not match up.

2. Intermediate Value Theorem

Use IVT to show a solution on [0,4] for f(x)= 2x^2 + 5x -3.

f(0)= 0 + 0 - 3= -3

f(4)= 32 + 20 - 3 = 49

 Since f is continuous on [0,4] and f(0)= -3<0<49 =f(4), then there exists c (element) [0,4]. 

Use IVT to show a solution on [2,4] for f(x)= -2x^2 - 5x + 3.

f(2)= -8 - 10 + 3 = -15

f(4)= -32 - 20 + 3 = -49

 Since f is continuous on [2,4] and f(2)= -49<0 but -49 is not less than 0 then it cannot be proved that there exists c (element) [2,4].

3. Types of Derivatives

Derivatives can be solved in two ways. The difference quotient

or the derivative in terms of slope.

Linear Derivative:

The derivative is -2.

The hardest part to finding a derivative is keeping track of the h's, or keeping track of what is being canceled.

4. Velocity

Instantaneous velocity is the slope of the tangent line at a certain point, but average velocity is the derivative that can be used to find the slope at any point.

LIMITLESS

1. Limits are the behavior of a function as it approaches an x-value from either side. 

2. When evaluating a limit, first try to plug in the number to see if it works. If the equation equals zero on the bottom, move to the next step.

If it is a square root function, rationalize the limit by multiplying the square root and opposite reciprocal of the constant with the original equation on both the numerator and denominator. Simplify. Then set the x to see if the limit exists. 

If it is a exponential function, factor it to cancel like terms. Simplify.

If it is two fractions, combine them by making the denominators the same. Simplify.

      4. In piecewise graphs the limit may not always exist. If the behavior of the graph from the left and the right do not come to the same point, and one does not exist, then the limit does not exist.

In this graph there is no limit at 1 because the behavior of both sides of the graph do not match up.

      5. Infinite limit: if the denominator is an extremely small integer, the graph will have an infinite limit, or asymptote. If one side of the graph goes to positive infinity while the other goes to negative infinity, the limit does not exist. If they both go in the same direction, the limit is infinite(either positive or negative). 

The graph will not reach 0 by a very small margin, the equation increasing until positive infinity.

Polynomial

y= 7x^3 - 11x^2 - 62x - 24

• The y-intercept can be found by looking at the constant term in the equation, or the number with no x by it. In this equation it's -24.
• To find out how many possible positive, negative, or imaginary solutions there are, use Descartes' Rule of Signs. Write the signs of the original equation and determine how many changes there are.  In this equation, (+ - - -) there is only one change. This means that there is one possible positive solution. The next step is to multiply the equation by -x, which means to change all of the odd exponents' coefficients will be opposite. So (- - + -), the new equation has 2 changes which means there are 2 possible negative or imaginary solutions. (Since imaginary numbers come in pairs.)
• The Rational Root Test provides all of the possible solutions to the problem. To find the rational roots take the constant term and put it over the leading coefficient (the coefficient before the highest degree). (-24/7) Take all of the possible factors of each number and keep them in the fraction. 1,2,3,4,6,8,12,24,-1,-2,-3,-4,-6,-8,-12,-24 / 1,7,-1,-7  This means that any of these solutions can be plugged into synthetic division to be proposed as a solution.
• Synthetic Division is the next step and to do it you must write the coefficient of each of the parts of the equation and the constant, including if there is a 0x. Place a factor you are testing on the outside of these numbers. The coefficients get dropped down and are multiplied by the number outside of the equation, starting from the first to the last. If the last number equals 0, the factor is a correct solution. Take the new numbers that developed from multiplication and apply them to a new rational root. If it does not equal 0, try again with another rational root.
  
• To check the solutions with your calculator, type the equation into the y= and where the line crosses the x-axis, those are your solutions. In this problem they are -2, 3/7, and 4.
• 
  

The 3 common trig triangles, their side lengths, and their sine, cosine, and tangent values.

Calculus Questions 1

1. The hardest topic in math so far is basically the end of math analysis. I don't remember any of it.

2. The topic that is the most fun is algebra because it's not too hard.

3. I want to go to college and have fun and learn and then live like a normal person.

4.  A school goal I have is to get a full ride scholarship to college. I can do that by getting all A's, managing my time well, and applying for schools and scholarships.

5. An out of school goal I have is to win the district championship and another game in football. I can do this by lifting weights, being enthusiastic in practice, and making good decisions when I play games.

6. A function is an equation where any x can be put into it and it will get exactly one y out of it.

         f(x)= x + 3         Graph: https://s3.amazonaws.com/grapher/exports/5kcn1oby6l.png